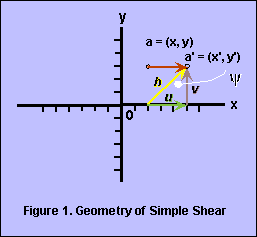
Simple shear like pure shear describes the changes of points in a body relative to each other, and therefore is described through an internal reference frame (Ferguson, 1994; Davis and Reynolds, 1996; van der Pluijm and Marshak, 1997). For example, consider some point (x, y) lying within a domain of potential simple shear with a coordinate system like that shown in Figure 1. After an increment of strain this point will be translated parallel to the x axis to some new position (x’, y’).
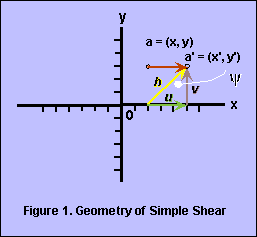
Now, note that the distance v is the amount that the point was translated parallel to the y axis, while u is the distance the point was translated parallel to the x axis. Vector h then is the resultant of these two components. From Figure 1 it should be obvious that v =h*cos(y ), and h = v/cos(y ). It therefore follows that u = v/cos(y ) * sin(y ) or upon simplifying u = v * tan(y ). However, by definition g = tan(y ). If y = v as demanded by the geometry of Figure 1, then u = g y . Putting these relationships in terms of coordinate transformations we then have
(1) x’ = x + gy,
and:
(2) y’ = 0x +1y.
Geologists refer to y as angular shear strain and to g as shear strain.
Equations (1) and (2) represent the algorithm utilized in Visual 2D Strain for transforming the coordinates of a circle to those of an ellipse for a given g . As shown in equation (3), equations (1) and (2) can be expressed in matrix format (Ferguson, 1994):
Geologists refer to the 2 X 2 matrix on the right hand side of equation (3), i.e.,
as the strain or transformation matrix for simple shear.